Newton's laws of motion
Level 0 (green)- this is basic material that you have probably encountered already, although the approach may be slightly different. No prior knowledge is assumed, but simple ideas of vectors are used.
These classical laws of motion tell us how to analyse and predict the motion of particles. They are an approximation from two points of view:
1. They break down at very high speeds (approaching the speed of light), where relativistic laws are applicable.
2. They break down at about the atomic scale, where quantum mechanical laws are applicable.
In chemistry we are rarely concerned with relativistic effects, but it is important to understand when classical mechanics is appropriate (for example the translational motion of a molecule) and when it is not (for example the motion of electrons within a molecule). You will gain this understanding as you proceed through the course.
This law tells us about what happens in the absence of forces.
Every body remains at rest or in a state of uniform motion in a straight line unless acted upon by an external force.
Any motion where the velocity is not constant or where the trajectory is not a straight line must therefore have a force acting on it, for example the circular motion of a conker whirled around on the end of a piece of string. The force on the conker acts through the string, and if the string is cut, the force is removed, and the conker flies off at a tangent.
A corollary to the first law is that a change in the velocity (i.e. an acceleration) is a response to the action of a force. The resistance to the force is measured by the mass (e.g. it is harder to accelerate a train than a ping-pong ball).
Newton's second law quantifies this observation.
Force is the rate of change of momentum.
Mathematically
![]()
Force is proportional to acceleration and is a vector in the same direction.
If more than once force acts on a body, the net force is the vector sum of all the forces operating, i.e. the resultant.
There are many examples of forces, and to apply the second law to a realistic situation the forces must be specified in a mathematical way.
Electrostatic force.
The most important force in chemical examples is the (Coulomb force) between two charges, q1 and q2 in a material of dielectric constant e.
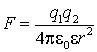
The force acts along the vector between the two charges.
A positive force indicates repulsion, a negative force attraction.
In a vacuum the dielectric constant is 1, but in condensed matter it may be very different from 1, for example in water at room temperature it is about 78, indicating that electrostatic forces are much weaker in water than in vacuum.
For more details see the tutorial on Electricity.
Gravitational force.
The gravitational force between two masses m1 and m2 is
![]()
The minus sign indicates attraction.
Gravity is too weak to be important as a force between molecules or constituents of molecules, however, the gravitational attraction of the earth is important in the local environment of experiments, for example in the measurement of pressure, and because the radius of the earth is so large compared to the small layer we live in, the gravitational formula can be simplified: if R is the radius of the earth and M its mass, then at a distance h above the surface
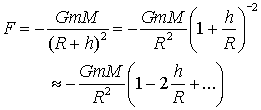
The second term in the binomial expansion is much smaller than 1, and so to this order the gravitational force is a constant, proportional to m, F = - mg.
Forces within molecules
The forces within molecules arise from a complicated combination of electrostatic interactions, and have a graph of the form
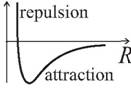
At short ranges the force is repulsive, tending to stretch the bond, at longer ranges the force is attractive, shortening the bond. The force is zero at the equilibrium bond length.
Forces between molecules have similar behaviour, although they are weaker, theiir origin is different and the detailed shape of the graph is different.
To every action there is an equal and opposite reaction.
This statement can be interpreted to mean that a force exerted by particle 1 on particle 2 is equal and opposite to the force exerted by particle 2 on particle 1.
So, for example, if there is an attractive force between particle 1 and particle 2 then the force on particle 1 is parallel to the vector leading from particle 1 to particle 2, and the force on particle 2 is equal in magnitude and opposite in direction, i.e. leading from particle 2 to particle 1.

Similarly, when a molecule collides with a surface the surface exerts a force on the molecule so that the molecule changes direction and bounces off the surface. There is an equal and opposite force on the surface. This is the molecular origin of gas pressure.

Consider a system of two particles. The only forces acting on these particles is the force of attraction or repulsion between them. The force on particle 1 is then equal and opposite to the force on particle 2.
![]()
Using Newton's second law, this implies that
![]()
in other words p1 + p2 is constant.
It is easy to generalise this idea to cases of several interacting particles.
If there is no net external force on a system, then the total resultant linear momentum of that system is constant.
This result is vital in the analysis of molecular collisions.